六邊形數
六邊形數是能排成正六邊形的多邊形數。第個六邊形數可用公式求得。其首十項為1, 6, 15, 28, 45, 66, 91, 120, 153, 190(OEIS:A000384)。第個六邊形數同時是第個三角形數。首個六邊形數之和可用公式求得。
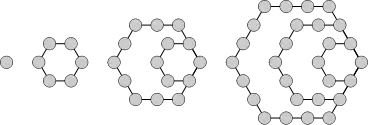
前四個六邊形數.
1 6 15 28
1830年勒讓德證明了任何大於1791的整數都能表達成最多4個六邊形數之和。
有13個正整數不能表達成4個六邊形數之和:5, 10, 11, 20, 25, 26, 38, 39, 54, 65, 70, 114, 130(OEIS:A007527)。
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.
